OVERLAP
More waves can exist in the same point of a mean in the same instant. For example, we can generate on a cord two inverse meeting impulses.
We see that these impulses pass one another and go on undisturbed.
Material bodies do not interpenetrate this way. A wave, however, is not a material body, but a series of movements of points that propagate in a material mean. Let’s now see what is the cord movement when two waves interpenetrate. If at a given time two or more waves overlap and are located at the same point, the movement of this point is the sum of movements that it would have with each wave separately. The interpenetration of one positive and one negative impulse creates during overlap a zero movement of the cord. The superposition principle applies both to the movement and the speed. That is, the actual vertical speed of each point on the cord is the sum of the speeds that it would separately have with each impulse. The overlap principle allows us to express a complex wave as a sum of simpler waves (sinusoidal waves).
THEOREM OF FOURIER
The characteristics of a sound are mainly three:
- Height, which makes us differentiate deep sounds from high-pitched ones and, as we have seen, is measured in Hz. For example, the "A" sample used for musical instruments intonation, is a sound wave with a frequency of 440Hz.<\li>
- Intensity allows us to appreciate the dynamics from pianissimo to fortissimo.<\li>
- Timbre, which allows us to distinguish the sounds of various musical instruments, even of the same height, which are in the orchestra and, as is often said, is the colour of a sound.<\li>
As for this last parameter, we will notice that the timbre is characterized by multiple factors sometimes dependent on others, such as by the acoustics of the listening room, the quality of the player you are using and so on. Parameters of the timbre can be reduced, for simplicity, in what are called the "attack transients" (which we'll see later) and the waveform. Up to now we have seen the simplest waveform: the sinusoidal one, which sound is certainly very annoying.
Selecting the button below, or the text, you can hear the sound of a sinusoid:
Sound of a sinusoid wave at 220 Hz
However, there are other periodic more complex waveforms (such as that of Figure 18) that, as the Fourier theorem states, can be expressed (and regenerated with electronic instruments) thanks to overlapping or sum of sinusoidal waves whose frequencies are whole multiples the periodic signal perceived as fundamental.
The waves that repeat their progression over time are called periodical (and thus in the graphical representation: their shape) in a quite regular shapes at length x intervals, while aperiodic are those waves that never repeat their progression over time. The spectrum of an aperiodic wave contains a continuous distribution of always-different component frequencies, while the spectrum of a periodic wave is unobtrusive.
It should be noted also that in some cases where it is not visually clear a periodicity of the waveform, we feel however that the acoustic sensation is that of a sound at an enough defined height.
Figure 17 shows a comparison between two waves: a periodical central piano C one, repeated in the image six times along the x axis and an aperiodic that has no repetition (blow sound). The links here below allow you to hear these two acoustic effects, of which the first that is more properly said sound, is tuned to 261.6 Hz, while the second which is more properly said noise, does not have a real intonation. The images were made with help of program Audacity, enlarging the waveform of a previously digitized signal.

Piano sound middle C note
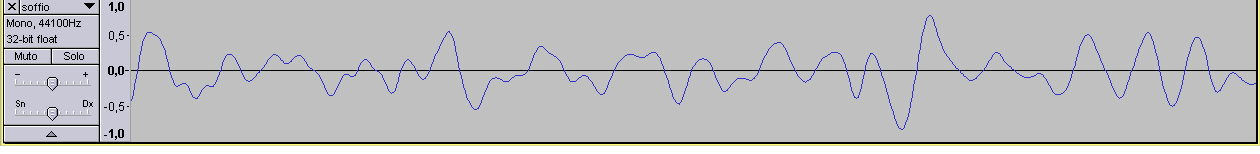
Noise by a whisker
